Quantum information processing promises algorithms surpassing their classical counterparts and unconditional secure of quantum key distributions. In those quantum information tasks, quantum entanglement plays a key role. The study of the role of entanglement in some physical systems such as condensed matter physics has also attracted much attention. On the one hand, quantum information has generated many alternative indicators of quantum phase transitions, on the other hand, it is expected that quantum phase transitions could possess operational significance with respect to the processing of quantum information.
　  Recently, Heng Fan with Ph.D student Jian Cui in Institute of Physics, Chinese Academy of Sciences, in collaboration with groups in Singapore, Brazil and UK, have reported that different quantum phases have different quantum computational power.
　  They show there exists quantum phase transitions that cause a distinct qualitative change in our ability to simulate certain quantum systems under perturbation of an external field by local operations and classical communication. In particular, by studying the general XY model they show that in certain quantum phases, the effect of adiabatic perturbations of the external magnetic field can be simulated by local spin operations, whereas the resulting effect within other phases results in fundamentally non-local interactions. They discuss the potential implications of such phase transitions to adiabatic quantum computation, where a computational advantage exists only when adiabatic perturbation of ground states results in multi-body interactions.
　  The method of this work is based on the local transformation of entangled states. It is shown that the necessary and sufficient condition for two bipartite states can be transformed from one to another is that a relationship should be satisfied between Renyi entropies of those two states. By applying this method to condensed matter models, it can be shown that different quantum phases can be distinguished clearly.
　  In summary, it is proposed that different quantum phases may have different computational power, this may provide hints on whether the physical systems are suitable for quantum information processing. This work was supported by 973 program and NSFC and other grants. The related work is published in Nature Communications 3, 812 (2012). 

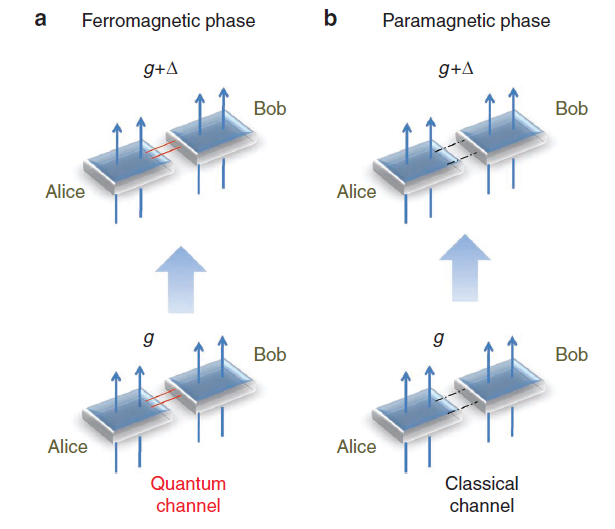Fig.1: Alice and Bob control one part of the ground state of the model. In one phase, by local operations and classical communication, Alice and Bob can change from one state to another. In another phase, a coherent transformation between Alice and Bob need be performed to change the ground state.（Image by Heng Fan et al.）

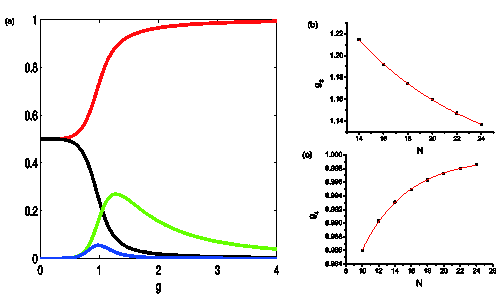Fig.2: Eigenvalues of the reduced density matrix of the ground state. （Image by Heng Fan et al.）

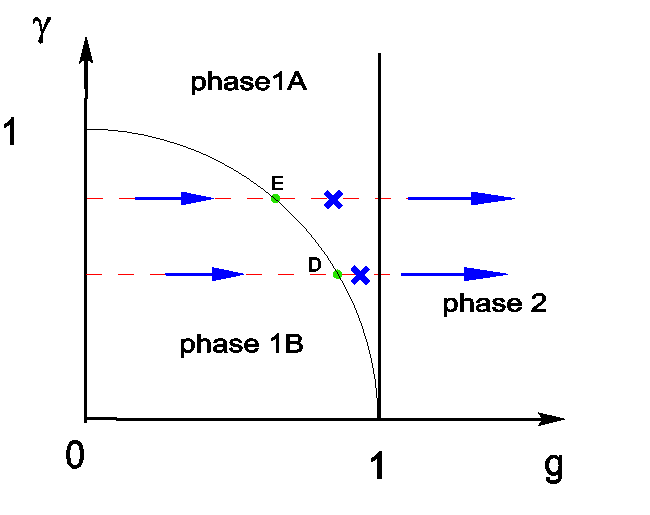Fig3:  Phase diagram of the XY model. （Image by Heng Fan et al.）

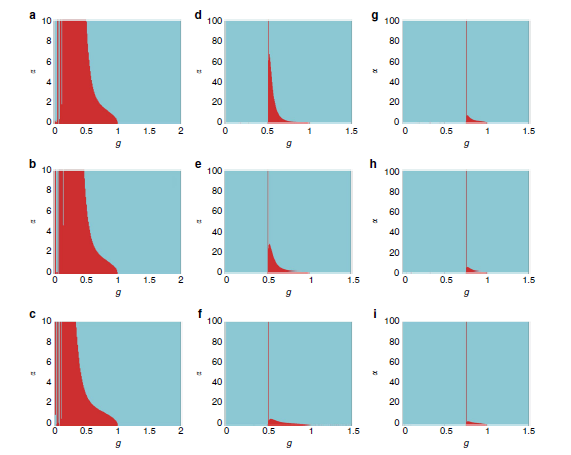Fig.4: Behaviors of Renyi entropies of the ground states. （Image by Heng Fan et al.）